ROBERVAL 1730

Description

Gilles Personne de Roberval, Divers ouvrages, Mémoires de l’Académie Royale des Sciences. Depuis 1666 jusqu’à 1699. Tome VI, Paris, Compagnie des Libraires, 1730

 A quarto volume (256×188 mm), (8)-712 pages and a folding plate. Contemporary full marbled calfskin binding, spine with five raised bands decorated and gilt bearing the title label, red edges.

 

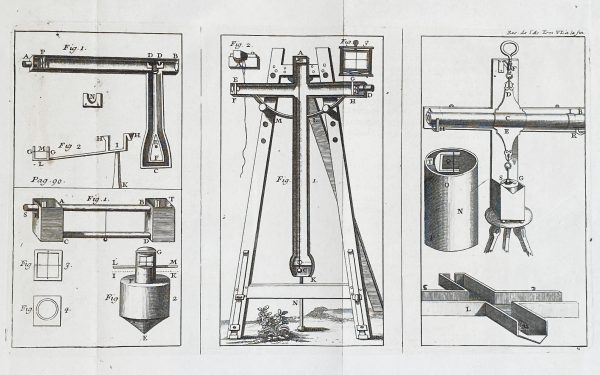
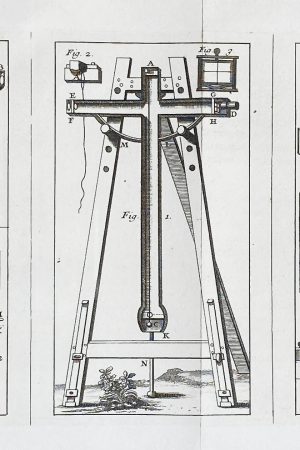
This volume of the Memoirs of the Royal Academy of Sciences contains articles by Gilles Personne de Roberval. French mathematician and physicist, he is the inventor of the balance with two beams known as the “balance of Roberval”. In 1666, he was one of the seven scholars who founded the Royal Academy of Sciences. He is less known for his work in mathematics, which is nevertheless important. Unfortunately, he tended to keep his discoveries secret, which led to controversy over the anteriority of some of his work.

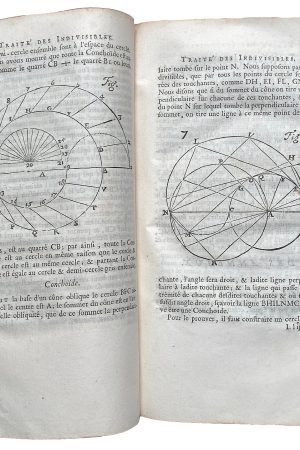
He thus developed the method of “Indivisibles”, but did not publish It and lost the honor of the discovery of integral calculus in favor of the independent work of Bonaventura Cavalieri. We also owe Roberval his work on cycloid curves and his observations on the composition of movements, making him “the founder of kinematic geometry” (DSB).

The present volume contains the following works which were published for the first time  in 1693 by the Imprimerie Royale:

– Observations on the composition of movements & on the means of finding the touching of curved lines.- Projet d΄un livre de Mecanique

– De Recognitione Aequarionum

– De Geometricâ Planarum & Cubicarum Aequationum resolutione

– Traité des indivisibles.

– De Trochoide ejusque spatio.

References: OHR [2327_1], DSB [(Roberval) XI, p. 487 :”Roberval’s tendency to keep his own discoveries secret has been attributed to his desire to profit from them in order to retain Ramus chair. But this habit also resulted in his tardy and frequent claims to priority. (…) Roberval was one of the leading proponents of the geometry of infinitesimals, which he claimed to have taken direcly from Archimedes, without having known the work of Cavalieri. (…). The numerous results that he obtain in this area are collected (…) under the title ‘ Traité des indivisibles ‘. One of the first important finding was, in modern terms, the definite integration of the rational power. (…).

On account of his method of the “composition of movements” Roberval may be called the founder of kinematic geometry”]